Tag: Programming

Posted on

Object-Oriented Programming (OOP)

OOP is based on “objects“. An object can perform a set of functions. The functions that the object performs defines the object’s behavior.

For example, the Batsman(object) can play shots or a Bowler(object) can Bowl.

Languages that support object-oriented programming have four important aspects:

1. Abstraction
2. Encapsulation
3. Inheritance
4. Polymorphism

Abstraction involves the process to define objects that represent abstract actions which can perform work,
report on and change their state, and interact with other objects in the system.

Encapsulation is the process of enclosing data and functions into a single element.

Inheritance is when an object or class is based on another object,
using parent objects properties to maintain the same behavior in the child object.

Polymorphism is the method of having a single interface to objects of different types.

Object-oriented programming (OOP) is a programming paradigm

Objects — > data — > fields — > attributes — > methods(code)

Some of the popular languages that support Object-Oriented Programming

Java, Python, C++, C#, Perl, Ruby , Objective-C, PHP, et al.

Posted on

Algorithms in Java

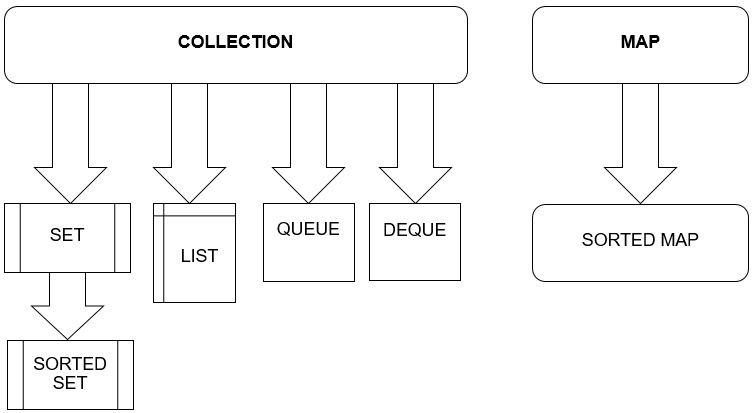
Most algorithms in Java operate on list instances and subsequently with other collection instances. The different algorithms used in Java are:

  • Sorting
  • Shuffling
  • Routine Data Manipulation
  • Searching
  • Composition
  • Finding Extreme Values

Sorting

The sort algorithm reorders a List so that its elements are in ascending order according to an ordering relationship. Two forms of the operation are provided.

FORM 1

The simple form takes a List and sorts it according to its elements’ natural ordering.

The sort operation uses a optimized merge sort algorithm which is fast and stable:

  • Fast: It is guaranteed to run in n log(n) time and runs substantially faster on nearly sorted lists. Empirical tests showed it to be as fast as a highly optimized quicksort. A quicksort is generally considered to be faster than a merge sort but isn’t stable and doesn’t guarantee n log(n) performance.
  • Stable: It doesn’t reorder equal elements. This is important if you sort the same list repeatedly on different attributes. If a user of a mail program sorts the inbox by mailing date and then sorts it by sender, the user naturally expects that the now-sequential list of messages from a given sender will (still) be sorted by mailing date. This is guaranteed only if the second sort was stable.

FORM 2

The second form of sort takes a Comparator in addition to a List and sorts the elements with the Comparator.

Shuffling

The shuffle algorithm does the opposite of what sort does, destroying any trace of order that may have been present in a List. That is, this algorithm reorders the List based on input from a source of randomness such that all possible permutations occur with equal likelihood, assuming a fair source of randomness. This algorithm is useful in implementing games of chance.

This operation has two forms:

One takes a List and uses a default source of randomness, and the other requires the caller to provide a Random object to use as a source of randomness.

Routine Data Manipulation

The Collections class provides five algorithms for doing routine data manipulation on List objects, as listed below:

  • reverse — reverses the order of the elements in a List.
  • fill — overwrites every element in a List with the specified value. This operation is useful for reinitializing a List.
  • copy — takes two arguments, a destination List and a source List, and copies the elements of the source into the destination, overwriting its contents. The destination List must be at least as long as the source. If it is longer, the remaining elements in the destination List are unaffected.
  • swap — swaps the elements at the specified positions in a List.
  • addAll — adds all the specified elements to a Collection. The elements to be added may be specified individually or as an array.

Searching

The binarySearch algorithm searches for a specified element in a sorted List. This algorithm has two forms.

  1. The first takes a List and an element to search for (the “search key”). This form assumes that the List is sorted in ascending order according to the natural ordering of its elements.
  2. The second form takes a Comparator in addition to the List and the search key, and assumes that the List is sorted into ascending order according to the specified Comparator. The sort algorithm can be used to sort the List prior to calling binarySearch.

The return value is the same for both forms.

Composition

The frequency and disjoint algorithms test some aspect of the composition of one or more Collections:

  • frequency — counts the number of times the specified element occurs in the specified collection.
  • disjoint — determines whether two Collections are disjoint; that is, whether they contain no elements in common.

Finding Extreme Values

The min and the max algorithms return, respectively, the minimum and maximum element contained in a specified Collection. Both of these operations come in two forms.

The simple form takes only a Collection and returns the minimum (or maximum) element according to the elements’ natural ordering.

The second form takes a Comparator in addition to the Collection and returns the minimum (or maximum) element according to the specified Comparator.

Source: The Java™ Tutorials

 

Posted on

Java Collection Framework

A collection or container is an object that groups multiple elements into a single unit, they are used to store, retrieve, manipulate and aggregate data.

Collections Framework

A collections framework is a unified architecture for representing and manipulating collections. All collections frameworks contain the following:

  • Interfaces: Interfaces are abstract data types that represent collections. Interfaces allow collections to be manipulated independently of the details of their representation. In object-oriented languages, interfaces generally form a hierarchy.
  • Implementations: These are the concrete implementations of the collection interfaces. Implementations are reusable data structures.
  • Algorithms: Algorithms are the methods that perform useful computations, such as searching and sorting, on objects that implement collection interfaces. The algorithms are said to be polymorphic: that is, the same method can be used on many different implementations of the appropriate collection interface. Algorithms possess reusable functionality.

Interfaces

The  collection interfaces encapsulate different types of collections. These interfaces allow collections to be manipulated independently of the details of their representation.

  • Collection — the root of the collection hierarchy. A collection represents a group of objects known as its elements. The Collection interface is the least common denominator that all collections implement and is used to pass collections around and to manipulate them when maximum generality is desired. Some types of collections allow duplicate elements, and others do not. Some are ordered and others are unordered. The Java platform doesn’t provide any direct implementations of this interface but provides implementations of more specific subinterfaces, such as Set and List.
  • Set — a collection that cannot contain duplicate elements.
  • SortedSet — a Set that maintains its elements in ascending order. Several additional operations are provided to take advantage of the ordering. Sorted sets are used for naturally ordered sets, such as word lists and membership rolls.
  • List — an ordered collection (sometimes called a sequence). Lists can contain duplicate elements. The user of a List generally has precise control over where in the list each element is inserted and can access elements by their integer index (position).
  • Queue — a collection used to hold multiple elements prior to processing. Besides basic Collection operations, a Queue provides additional insertion, extraction, and inspection operations.

Queues usually order elements in a FIFO (first-in, first-out) manner.

  • Deque — Similar to queue but Deques can be used both as FIFO (first-in, first-out) and LIFO (last-in, first-out). In a deque all new elements can be inserted, retrieved and removed at both ends.
  • Map — an object that maps keys to values. A Map cannot contain duplicate keys; each key can map to at most one value.
  • SortedMap — a Map that maintains its mappings in ascending key order. This is the Map analog of SortedSet. Sorted maps are used for naturally ordered collections of key/value pairs, such as dictionaries and telephone directories.

If an unsupported operation is invoked, a collection throws an UnsupportedOperationException.

Note:  All core collection interfaces are generic.

Source: The Java™ Tutorials